Spell Test PLAY Match Gravity Commutative Property of Addition Click card to see definition 👆 Tap card to see definition 👆 x y= y xIf x x x and y y y are real numbers such that x y = 7 xy=7 x y = 7 and x 3 y 3 = 133 x^3y^3=133 x 3 y 3 = 1 3 3, find the value of x y xy x y Submit your answer 3 3 ( 1640 ) 1 3 \sqrt{\sqrt{\sqrt3{\color{#3D99F6}{}} {\sqrt3{\color{#3D99F6}{} \color{teal}{3(1640) 1}}}}} 3 6 4 0 0 0 3 6 4 0 0 0 3 ( 1 6 4 0 ) 1Here, Right hand side = Left hand side which means that (a3) (a3) is an identity Using Activity Method In this method, the algebraic identity is verified geometrically by taking different values of a x and y In the activity method, the identities are verified by cutting and pasting paper

Solved Prove The Identity Simplify At Each Step 3 Cos X Chegg Com
(x+y)^3 identity class 9
(x+y)^3 identity class 9-X = 2 y = 2 # and print (x > 0 and y < 0) # True # or print (x > 0 or y < 0) # True # not print (not (x > 0 and y < 0)) # False Identity Operators Identity operators are used to check if two objects point to the same object, with the same memory location Ex 25, 9Verify (i) x3 y3 = (x y) (x2 – xy y2)LHS x3 y3We know (x y)3 = x3 y3 3xy (x y)So, x3 y3 = (x y)3 – 3xy (x y) = (x y)3 – 3xy




Learn Algebraic Identity Of X Y And X Y In 3 Minutes
Who are the experts?X y x' x'y xx'y xy 0 0 1 0 0 0 0 1 1 1 1 1 1 0 0 0 Stack Exchange Network Stack Exchange network consists of 179 Q&A communities including Stack Overflow , the largest, most trusted online community for developers to learn, share their knowledge, and build their careersExample 3 Jeremy uses the polynomial identity (x² – y?)² (2xy)² = (x² y²)² to generate the Pythagorean Triple, 9, 40, 41 What values of x and y did he use to generate the values for the three sides of a right triangle?
Since x − y = 3 xy=3 x − y = 3 implies y = x − 3, y=x3, y = x − 3, substituting this into the given identity gives a x (x − 3) b x c (x − 3) 9 = 0 a x 2 (− 3 a b c) x − 3 (c − 3) = 0 \begin{aligned} ax(x3)bxc(x3)9&=0\\ ax^2(3abc)x3(c3)&=0 \end{aligned} a x (x − 3) b x c (x − 3) 9 a x 2 (− 3 a b c) x − 3 (c − 3) = 0 = 0Use the distributive property to multiply x y by x 2 − x y y 2 and combine like terms x^ {3}y^ {3}=x^ {3}y^ {3} x 3 y 3 = x 3 y 3 Subtract x^ {3} from both sides Subtract x 3 from both sides x^ {3}y^ {3}x^ {3}=y^ {3} x 3 y 3 − x 3 = y 3 Combine x^ {3} and x^ {3} to get 0Algebra Formulas for Class 9 Algebra is a branch of mathematics in which universal symbols and letters are used to represent quantities and numbers in equations and formulas Algebra is divided into two sections elementary algebra and modern algebra (Abstract algebra) Algebra formulas for Class 9 comprise formulas relating to algebra identities or expressions
Polynomial Identities When we have a sum (difference) of two or three numbers to power of 2 or 3 and we need to remove the brackets we use polynomial identities (short multiplication formulas) (x y) 2 = x 2 2xy y 2 (x y) 2 = x 2 2xy y 2 Example 1 If x = 10, y = 5a (10 5a) 2 = 10 2 2·10·5a (5a) 2 = 100 100a 25a 2Prove the identity 3 cos (x y) 3 cos (x y) = 6 cos (x) cos (y) Use a Reciprocal Identity, and then rewrite as a single rational expression 7 tan (x) 7 tan (y) = 7 sin (y) cos (y) cos (x) = cos (x) cos (y) Use an Addition or Subtraction Formula to simplify Prove the identity sin ( 1The two polynomials are called as special binomials because the first term in each expression is same Hence, the product of them is called as the special product of binomials It is written in the following mathematical form algebraically ( x − a) ( x − b) The product of them is equal to an algebraic expression x 2 − ( a b) x a b




Adidas X Y 3 Shiku Run Black Creme Fx1416 Garmentory




Expand Each Of The Following Using Suitable Identities 2x 5y 3z 2
This identity can also be expressed as, This is the required standard algebraic identity In the expression, if we replace ywith (−y), we will get the identity x3−y3 Now, let's further verify this numerically with an example To verify, let's take the values for xand yand put inTherefore, x=50, and y=3 I do not get thisAnswer 1 There are two words that start with a, two that start with b, two that start with c, for a total of \(222\text{}\) Answer 2 There are three choices for the first letter and two choices for the second letter, for a total of \(3 \cdot 2\text{}\) Since the two answers are both answers to
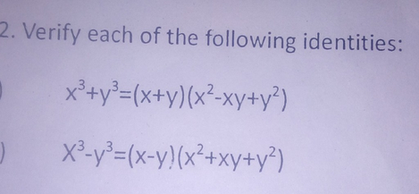



2 Verify Each Of The Following Identities X3 Y3 X Y X2 X Scholr




Expand Each Of The Following Using Suitable Identities
It is read as $x$ plus $y$ whole cube It is mainly used in mathematics as a formula for expanding cube of sum of any two terms in their terms ${(xy)}^3$ $\,=\,$ $x^3y^33x^2y3xy^2$ Proofs The cube of $x$ plus $y$ identity can be proved in two different mathematical approaches Algebraic method Learn how to derive the expansion of cube of $x$ plus $y$ identity by the For $x,y$ in G, prove that $x = y =e$ if $xy^2=y^3x $ and $yx^2=x^3y$ where $e$ is the identity element in G I have filled pages trying to solve but unable to reach Find the value of 53^3 using the identity (xy)^3=x^33x^2y3xy^2y^2 Hint 53^3 =(503)^3;



Identity Mathematics Wikipedia



Rational Functions And Endomorphisms Separable Endomorphisms Parallelogram Identity And Degree Map
Solution (x 3 8y 3 27z 3 – 18xyz)is of the form Identity VIII where a = x, b = 2y and c = 3z So we have, So we have, (x 3 8y 3 27z 3 – 18xyz) = (x) 3 (2y) 3 (3z) 3 – 3(x)(2y)(3z)= (x 2y 3z)(x 2 4y 2 9z 2 – 2xy – 6yz – 3zx)(xyz)^3 put xy = a (az)^3= a^3 z^3 3az ( az) = (xy)^3 z^3 3 a^2 z 3a z^2 = x^3y^3 z^3 3 x^2 y 3 x y^2 3(xy)^2 z 3(xy) z^2 =x^3 y^3 z^3 3 x^2y 3xy^2 3 ( x^2 y^2 2xy ) z 3x z^2 3yz^2 =x^3y^3z^3 3x^2 y3xy^2 3x^2 z 3y^2 z 6xyz 3xz^2 3 yz^2 arrange in orderTherefore, x = 50 and y = 3 I dont get this at all?




How To Expand Using The Identity X Y 3 X3 Y3 3x2y 3xy2 Youtube



1
We can find the value by using the trigonometric identity of sin 3 x Answer The formula of Sin 3 x is (3 sinx sin3x)/4 Let's proceed step by step Explanation As per trigonometric identity, we have sin 3x = 3sin x 4sin 3 x On transposing terms, 4 sin 3 x = 3sinx sin 3x Divide both sides of the equation 4 sin 3 x = 3sinx sin 3x by The formula is (xy)³=x³3x²y3xy²y³ You convert it to (xy)³=x³3xy(xy)y³ for an illustration You take the drawing of the formula (xy)³=x³3x²y3xy²y³ from above and replace a by the difference xyExperts are tested by Chegg as specialists in their subject area We review their content and use your feedback to keep the quality high Transcribed image text Prove the identity 3 cos (x y) 3 cos (x y) = 6 cos (x) cos (y)
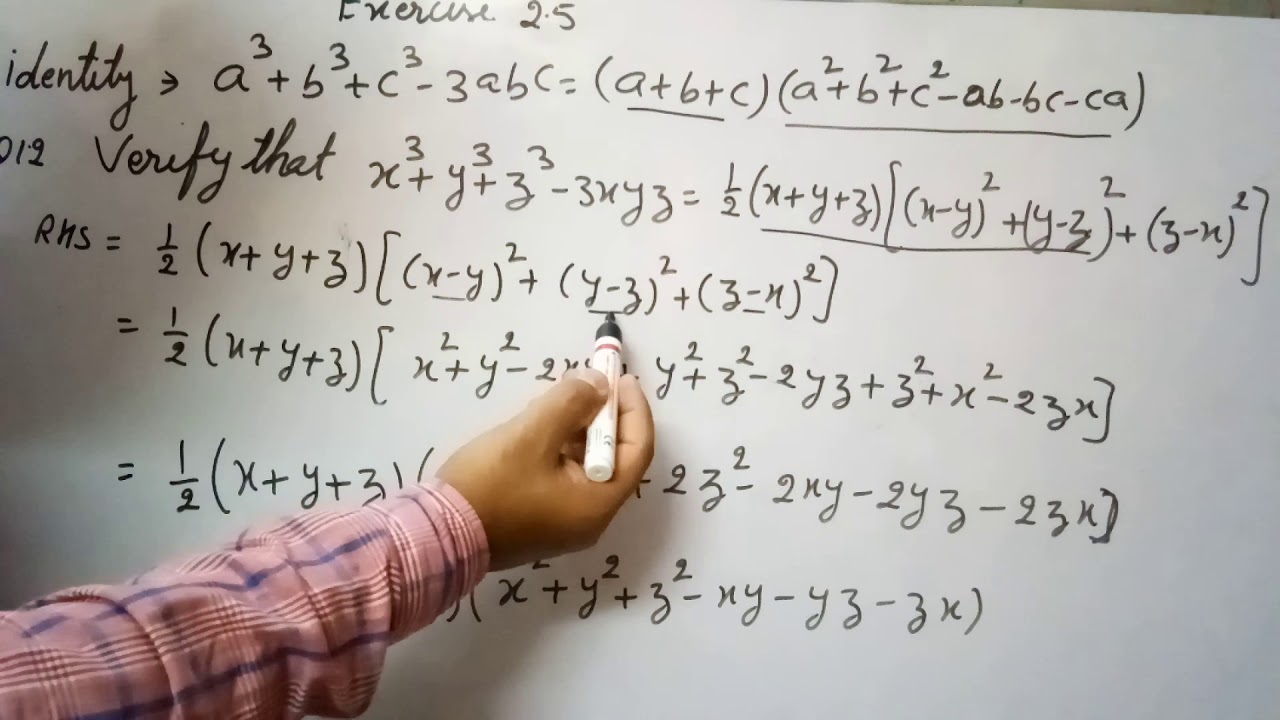



Ex 2 5 Q No 12 Verify X3 Y3 Z3 3xyz 1 2 X Y Z X Y 2 Y Z 2 Z X 2 Youtube
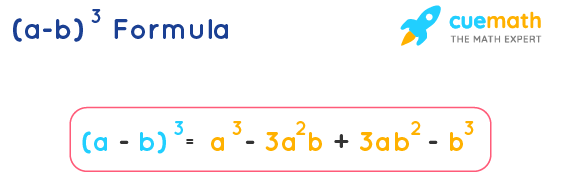



A Minus B Whole Cube Formula Examples A B 3 Formula



0 件のコメント:
コメントを投稿